Question

A nonconducting sphere has radius R = 1.81 cm and uniformly distributed charge q = +2.08 fC. Take the electric potential at the sphere's center to be V0 = 0. What is V at radial distance from the center (a) r = 1.20 cm and (b) r = R? (Hint: See an expression for the electric field.)

Answer 1

a) V = -0.227 mV

b) V = -0.5169 mV

Step-by-step explanation:

a)

Inside a sphere with a uniformly distributed charge density, electric field is radial and has a magnitude

E = (qr) / (4πε₀R³)

As we know that

V = -
`\int\limits^r_0 {E} \, dr`

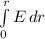
By solving above equation, we get

V = (-qr²) / (8πε₀R³)

When

R = 1.81 cm

r = 1.2 cm

q = +2.80 fC

ε₀ = 8.85 × 10⁻¹²

V = (-2.80 × 10⁻¹⁵ × (1.2 × 10⁻²)²) / (8 × 3.14 × 8.85 × 10⁻¹² × (1.81 × 10⁻²)³)

V = -2.27 × 10⁻⁴ V

V = -0.227 mV

b)

When

r = R

R = 1.81 cm

q = +2.80 fC

ε₀ = 8.85 × 10⁻¹²

V = (-qR²) / (8πε₀R³)

V = (-q) / (8πε₀R)

V = (-2.80 × 10⁻¹⁵) / (8 × 3.14 × 8.85 × 10⁻¹² × (1.81 × 10⁻²))

V = -5.169 × 10⁻⁴ V

V = -0.5169 mV