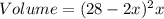Question

From a 28-inch by 28-inch piece of metal, squares are cut out of the four corners so that the sides can then be folded up to make a box. Let x represent the length of the sides of the squares, in inches,that are cut out. Express the volume of the box as a function of x.

Answer 1

Answer:t The volume of the box as a function of x is given by

`Volume=(28-2x)^2x`

Explanation:

Since we have given that

Length of metal = 28 inch

Width of metal = 28 inch

Since squares are cut out of the four corners so that the sides can then be folded up to make a box.

Let the length of the sides of the squares that are cut out be 'x'.

So, Length of box would be

`28-2x`

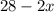
Width of the box would be

`28-2x`

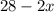
Height of the box would be x.

As we know the formula for "Volume of box":

`Volume=(28-2x)(28-2x)x\\\\Volume=(28-2x)^2x`

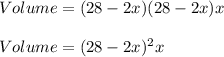
Hence, the volume of the box as a function of x is given by

`Volume=(28-2x)^2x`