Answer:
a) t = 1.47 h b) t = 1.32 h
Step-by-step explanation:
a) In this problem the plane and the wind are in the same North-South direction, whereby the vector sum is reduced to the scalar sum (ordinary). Let's calculate the total speed
v =
 f -
f -
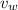
v = 585 -32.1
v = 552.9 km / h
We use the speed ratio in uniform motion
v = x / t
t = x / v
t = 815 /552.9
t = 1.47 h
b) We repeat the calculation, but this time the wind is going in the direction of the plane
v=
 f -
f -
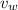
v 585 + 32.1
v = 617.1 km / h
t = 815 /617.1
t = 1.32 h