Answer:
a. The line segment is vertical and lies in 2nd and 3rd quadrant.
b. The length of the segment is 8 units.
Explanation:
We have been given the end points of a line segment.
a. The given coordinates are (−3, 3) and (−3, −5). We can see that x-coordinates of both points are same, which means that the line segment is vertical, which passes the x-axis at
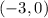 .
.
Since the x-coordinates of both endpoints are negative and y-coordinates are negative and positive, therefore, the line segment lies in 2nd and 3rd quadrant.
b. Since the x-coordinates of both endpoints are same, so the length of the line segment would be difference between the y-coordinates.

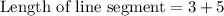
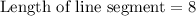
Therefore, the length of the line segment is 8 units.