Answer:
Isolate the variable by dividing each side by factors that do not contain the variable.
After solving the inequality for x, the solution written in Inequality Form would be:
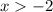
or
The solution written in Interval Notation would be: (-2, ∞)
_____________________________________________________
"What is Interval Notation?"
An easy way of understanding what interval notation would be is that it is a way of writing subsets of the real number line. When writing in Interval Notation, you would use closed brackets or parentheses most often.
"What is Inequality Form?"
Within mathematics, an inequality is known as a relation in which makes a non-equal comparison between two number or any other mathematical expressions. In other words, it is most of the time used to compare two numbers on the number line based on their size. So, when someone asks you to write in Inequality Form, it most likely means that they want you to convert any given information prior to the mathematical question into an inequality.
Hope this kind of helps! If not, feel free to comment below and I'll see what else I can do to help. Thanks and good luck!