Question

Dr. John Paul Stapp was a U.S. Air Force officer who studied the effects of extreme deceleration on the human body. On December 10, 1954, Stapp rode a rocket sled, accelerating from rest to a top speed of 282 m/s (1015 km/h) in 5.00 s, and was brought jarringly back to rest in only 1.40 s! Calculate his acceleration and deceleration. Express each in multiples of g (9.80 m/s2) by taking its ratio to the acceleration of gravity.

Answer 1

(a) Acceleration = 5.755 g

(b) Deceleration = 20.55 g

Step-by-step explanation:

We have given that rocket accelerates from rest to 282 m/sec

So initial velocity u = 0 m/sec

And final velocity v = 282 m/sec

And time t = 5 sec

From first equation of motion v = u+at

So acceleration
`a=(v-u)/(t)=(282-0)/(5)=56.4 m/sec^2`

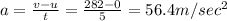
We know that g = 9.8
`m/sec^2`

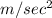
So 56.4
`m/sec^2` =
`=(56.4)/(9.8)=5.755g`

(b) Now initial velocity u = 282 m/sec

And finally it comes to rest so final velocity v = 0 m/sec

Time t = 1.4 sec

So acceleration a
`=(v-u)/(t)=(0-282)/(1.4)=-201.428m/sec^2`

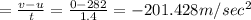
So deceleration = 201.428
`m/sec^2`

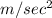
We know that g = 9.8
`m/sec^2`

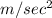
So 201.428
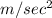`m/sec^2` =
`=(201.428)/(9.8)=20.55g`