Answer:
We fail to reject the null hypothesis because -1.7678 does not fall inside the rejection region z < -1.96 or z >1.96
Explanation:
We have that the machine will fill bottles according to a normally distributed process, let's say
 is a random variable that represents this process. We know that
is a random variable that represents this process. We know that
 has a mean of 47 fluid ounces and a standard deviation of 0.4 fluid ounces. We have a sample of n = 50 bottles and the observed value
has a mean of 47 fluid ounces and a standard deviation of 0.4 fluid ounces. We have a sample of n = 50 bottles and the observed value
 .
.
We want to test
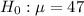 vs
vs
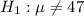 (two-tailed alternative)
(two-tailed alternative)
We have n = 50 large enough, and the point estimator of the mean
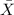 , which is normally distributed with the same mean than
, which is normally distributed with the same mean than
 , i.e.,
, i.e.,
 , and with standard deviation given by
, and with standard deviation given by
 . Therefore, our test statistic is
. Therefore, our test statistic is
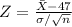 and
and
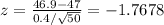 .
.
As we want a significance level of 0.05, we should find the values
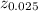 and
and
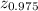 , i.e., the 2.5th quantile and the 97.5th quantile for the standard normal distribution. These values are -1.96 and 1.96 respectively. The rejection region is given by z . We fail to reject the null hypothesis because -1.7678 does not fall inside the rejection region.
, i.e., the 2.5th quantile and the 97.5th quantile for the standard normal distribution. These values are -1.96 and 1.96 respectively. The rejection region is given by z . We fail to reject the null hypothesis because -1.7678 does not fall inside the rejection region.