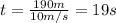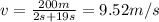Answer:
a) 10m
b)
i)
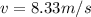
ii)
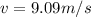
iii)
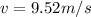
Step-by-step explanation:
The first 2.0s the sprinter maintains a constant acceleration. So we have to use the Constant acceleration motion formulas to solve the first part of the movement, then we need to use the contant velocity motion formulas for the rest of the movement.
a)

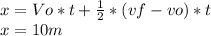
b)
the sprinter run 10 meters with constant accelaration then it start a constant velocity movement, so:
i) x=50-10=40m
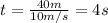

ii) x=100-10=90m


iii) x=200-10=190m