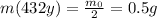Question

Americium-241 is a radioactive substance used in smoke detectors. The half life of americium is 432 years. If a smoke detector initially contains 1 gram of Americium 241, how much will remain in 432 years?

Answer 1

0.5 g

Step-by-step explanation:

The half-life of a radioactive isotope is the time it takes for a certain amount of that isotope to halve.

In this case, we have an isotope of Americium-241, which has a half-life of

`\tau = 432 y`

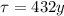
This means that after 1 half-life (432 years), there will be an amount of americium equal to half its initial amount.

In this problem, the initial amount of americium is

`m_0 = 1 g`

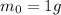
Therefore, after 432 years (1 half-life), the amount of americium left will be half:

`m(432y) = (m_0)/(2)=0.5 g`