Question

If the vertex of a parabola is (3,11) and another point on the curve is (2,7),

what is the coefficient of the squared expression in the parabola's equation?

Answer 1

`\large \boxed{-4}`

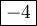
Explanation:

The vertex form of the equation for a parabola is

y = a(x - h)² + k

where h and k are the coordinates of the vertex

and a is the coefficient of x² in the standard form.

Data:

Vertex at (3,11)

A point at (2,7)

Calculations:

1. Substitute the coordinates of the vertex into the equation

y = a(x - 3)² + 11

2. Substitute the coordinates of the point into the equation and solve for a

`\begin{array}{rcl}7 & = & a(2 - 3)^(2) + 11\\7 & = & a(-1)^(2) + 11\\7& = &a + 11\\a & = & \mathbf{-4}\\\end{array}\\\text{The coefficient of x$^(2)$ in the parabola's equation is $\large \boxed{\mathbf{-4}}$}`