Answer: the correct option is
(D) The imaginary part is zero.
Step-by-step explanation: Given that neither a nor b are equal to zero.
We are to select the correct statement that accurately describes the following product :

We will be using the following formula :
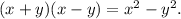
From product (i), we get
![P\\\\=(a+bi)(a-bi)\\\\=a^2-(bi)^2\\\\=a^2-b^2i^2\\\\=a^2-b^2* (-1)~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~[\textup{since }i^2=-1]\\\\=a^2+b^2.](https://img.qammunity.org/2020/formulas/mathematics/middle-school/4mrszwib3vgttdb8n1fmcm0fw8qtmpe7f2.png)
So, there is no imaginary part in the given product.
Thus, the correct option is
(D) The imaginary part is zero.