Question

An IQ test is designed so that the mean is 100 and the standard deviation is 18 for the population of normal adults. Find the sample size necessary to estimate the mean IQ score of statistics students such that it can be said with 95​% confidence that the sample mean is within 3 IQ points of the true mean. Assume that sigmaσequals=18 and determine the required sample size using technology. Then determine if this is a reasonable sample size for a real world calculation.

The required sample size is ____? (round up to the nearest integer).
Is this a reasonable sample size for a real world calculation? ______.

Answer 1

n = 139

It isnt reasonable for a real world calculation

Explanation:

The error for the true mean is calculated as follows:

`E= z(\sigma)/(√(n))`

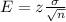
the z score for a 95% confidence is 1.96, replacing in the formula above you get:

`3 = 1.96(\mu)/(√(n) )`

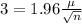
Solving for n you get that n = 138.3 = 139

According to (Yamane, 1967) for populations bigger than 100,000 you need a sample size of 1,111