Question

Periodic interest rates. You have a savings account in which you leave the funds for one year without adding to or withdrawing from the account. Which would you rather​ have: a daily compounded rate of 0.040​%, a weekly compounded rate of 0.285​%, a monthly compounded rate of 1.35​%, a quarterly compounded rater of 3.50​%, a semiannually compounded rate of 8.5​%, or an annually compounded rate of 18​%? What is the effective annual rate​ (EAR) of a daily compounded rate of 0.040​%?

Answer 1

We will pick the highest rate, which is 18% annually

effective rate of 0.04% compounded daily: 15.72%

Step-by-step explanation:

we will calculate the equivalent rate for each alternative:

daily: 0.040%

`(1+ r)^(time) = 1+ r_e`

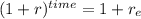
time 365.00 (365 days per year)

rate 0.040% = 0.04/100 = 0.00040

`1 \: (1+ 0.0004)^(365) = 1 + r_e`

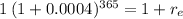
effective: 0.1572 = 15.72%

weekly 0.285

there are 52 week per year

`1 \: (1+ 0.00285)^(52) = 1 + r_e`

effective rate: 0.15950 = 15.95%

monthly 1.35%

12 months per year

`1 \: (1+ 0.0135)^(12) = 1 + r_e`

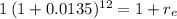
effective rate = 0.17459 = 17.46%

quarterly: 3.5%

four quarter per year

`1 \: (1+ 0.035)^(4) = 1 + r_e`

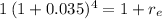
effective rate = 0.14752 = 14.75%

semiannually 8.5%

dos half

`1 \: (1+ 0.085)^(2) = 1 + r_e`

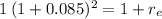
effective rate = 0.17723 = 17.72%

annual of 18%

We will pick the highest rate, which is 18% annually