Question

Calculat the sideral period of Mars given its synodic period is 780 days. 7. Calculate the synodic period of Venus given its sidereal period is 229 days.

Answer 1

686 days for sideral period of Mars.

615 days for synodic period of Venus.

Step-by-step explanation:

The equations we need to use is:

`(1)/(P)=(1)/(E)\pm(1)/(S)`

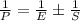
Where P is the sidereal period, S the synodic period and E the Earth's period (365 days) and where the + is used for inferior planes (Venus) and the - for superior ones (Mars).

For the sidereal period of Mars we then have:

`P_M=(1)/((1)/(E)-(1)/(S_M))=(1)/((1)/(365days)-(1)/(780days))=686days`

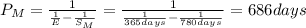
And for the synodic period of Venus we then have:

`S_V=(1)/((1)/(P_V)-(1)/(E))=(1)/((1)/(229days)-(1)/(365days))=615days`

(Commonly for Venus a sidereal period of 225 days is given, which changes this result to 587 days).