Question

A hollow sphere (of negligible thickness) with no load, with a radius of 8.00 cm, surrounds a charge of 20.00 μC located at the origin of a Cartesian coordinate system. A 2.00 mm radius drill is aligned along the Z axis and a hole is made on the surface of the sphere. Calculate the electrical flow through the borehole.

a

39.3 N*m2/C

b

17.7 N*m2/C

c

587 N*m2/C

d

35.3 N*m2/C

e

353 N*m2/C

Answer 1

The electrical flow through the borehole is 353 Nm²/C.

(e) is correct option.

Step-by-step explanation:

Given that,

Radius = 8.00 cm

Charge = 20.00 μC

radius of drill = 2.00 mm

We need to calculate the electrical flow through the borehole

Using formula of electric flow

`\phi=EA`

`\phi=(kq)/(R^2)*\pi* r^2`

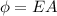
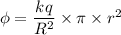
Where, q = charge

R = radius of sphere

r = radius of drill

Put the value into the formula

`\phi=(9*10^(9)*20*10^(-6))/((8*10^(-2))^2)*\pi*(2.00*10^(-3))^2`

`\phi=353\ Nm^2/C`

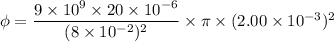
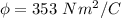
Hence, The electrical flow through the borehole is 353 Nm²/C.