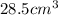Question

What is the volume V of a sample of 4.00 mol of copper? The atomic mass of copper (Cu) is 63.5 g/mol, and the density of copper is 8.92 x 10^3kg/m^3. Express your answer in cubic centimeters to 3 significant figures.

Answer 1

Answer:
`V=28.4* 10^(-6) m^3`

Step-by-step explanation:

Given

no of moles=4

Atomic mass of copper=63.5 g/mol

Density of copper
`=8.92* 10^3 kg/m^3`

no of moles
`=(mass of copper)/(Atomic weight)`

`4=(mass)/(63.5)`

mass=254 gm

and
`mass=density* volume`

`254* 10^(-3)=8.92* 10^3* V`

`V=(254* 10^(-3))/(8.92* 10^3)`

`V=28.4* 10^(-6) m^3`

Answer 2

Answer : The volume of a sample of 4.00 mol of copper is
`28.5cm^3`

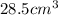
Explanation :

First we have to calculate the mass of copper.

`\text{ Mass of copper}=\text{ Moles of copper}* \text{ Molar mass of copper}`

`\text{ Mass of copper}=(4.00moles)* (63.5g/mole)=254g`

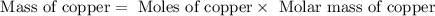
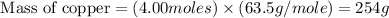
Now we have to calculate the volume of copper.

Formula used :

`Density=(Mass)/(Volume)`

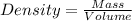
Now put all the given values in this formula, we get:

`8.92* 10^3kg/m^3=(254g)/(Volume)`

`Volume=(254g)/(8.92* 10^3kg/m^3)=2.85* 10^(-2)L=2.85* 10^(-2)* 10^3cm^3=28.5cm^3`

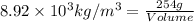
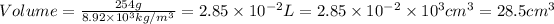
Conversion used :

`1kg/m^3=1g/L\\\\1L=10^3cm^3`

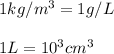
Therefore, the volume of a sample of 4.00 mol of copper is
`28.5cm^3`