Question

A girl, standing on the roof of a house which is 5.00 m high, throws a stone vertic upward. It strikes the ground 7.00 s after being thrown. Calculate: The initial velocity of the stone The maximum height the stone reaches above a. b. i. The roof ii. The ground and c.

Answer 1

Step-by-step explanation:

Given

Height of roof=5 m

Time taken by stone to reach ground 7 s

Let u be the initial velocity

therefore maximum height is h

`v^2-u^2=2as`

`0-u^2=2(-g)h`

`u=√(2gh)`

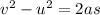
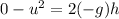
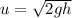
time taken to reach max height

v=u+at

0=u-gt

`t=(u)/(g)`

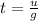
Now time taken to reach ground is
`t_2`

`h+5=0* t_2+(gt_2^2)/(2)`

`(u^2)/(2g)+5=(gt_2^2)/(2)`

`t_2=\sqrt{(u^2+10g)/(g^2)}`

`t_2=(√(u^2+10g))/(g)`

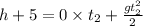
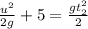
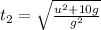
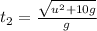
total time is
`t+t_2=7`

`(u)/(g)+(√(u^2+10g))/(g)=7`

`(√(u^2+10g))/(g)=7-(u)/(g)`

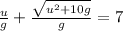
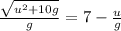
Squaring both side

`u^2+10g=49g^2+u^2-14ug`

`14u=49g-10`

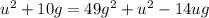
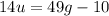
u=33.58 m/s

therefore
`h=(u^2)/(2g)`

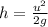
h=57.55 m

maximum height from ground is 57.55+5=62.55 m