Question

An archer fires and arrow while standing atop a 5.15 m tall wall. The arrow is fired at an angle of 55 degrees and has a launch velocity of 52.4 m/s. (This is the actual launch velocity for English Longbows!) a. What is the time of flight for the arrow?

b. How far from the wall does the arrow strike the ground?

Answer 1

Step-by-step explanation:

Given

height of wall=5.15 m

angle of launch
`(\theta )=55^(\circ)`

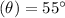
Launch velocity(u)=52.4 m/s

Time of flight will be sum of time of flight of projectile+time to cover 5.15 m

Time of flight of arrow
`=(2usin\theta )/(g)`

`t=(2* 52.4* sin55)/(9.81)=8.76 s`

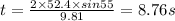
Now time require to cover 5.15 m

Here at the time of zero vertical displacement of arrow i.e. when arrow is at the same height as of building then its vertical velocity will change its sign compared to initial vertical velocity.

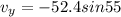`v_y=-52.4sin55` at zero vertical displacement

Thus time required will be
`t_2`

`5.15=52.4sin55* t+(gt^2)/(2)`

`4.9t^2+42.92t-5.15=0`

`t=0.118 s i.e. t_2=0.118 s`

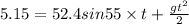
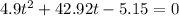
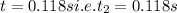
total time =
`t_1+t_2=8.76+0.118=8.878 s`

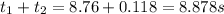
(b)Horizontal distance=Range of arrow(R_1) + horizontal distance in 0.118 s
`(R_2)`

`R_1=(u^2sin2\theta )/(g)=(52.4^2* sin110)/(9.8)=263.28 m`

`R_2=52.4cos55 * 0.118=3.546 m`

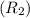
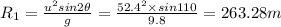
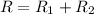`R=R_1+R_2`=263.28+3.546=266.82 m