Question

A particle has rest mass 10-30 kg and travels at 0.5 c with respect to the Laboratory. Find its inertial mass, its momentum, its kinetic energy and its total energy

Answer 1

Step-by-step explanation:

It is given that,

The rest mass of the particle,
`m_o=10^(-30)\ kg`

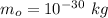
Speed of the particle, v = 0.5c

Firstly calculating the relativistic factor. The formula is as follows :

`\gamma=\frac{1}{\sqrt{1-(v^2)/(c^2)}}`

`\gamma=\frac{1}{\sqrt{1-((0.5c)^2)/(c^2)}}`

`\gamma=1.032`

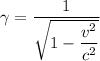
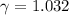
1. Inertial mass is given by :

`m=\gamma * m_o`

`m=1.032 * 10^(-30)`

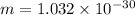
2. The momentum is given by :

`p=\gamma* mv`

`p=1.032* 1.032 * 10^(-30)* 0.5c`

`p=1.59* 10^(-22)\ kg-m/s`

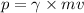
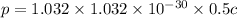
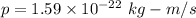
3. Kinetic energy of the particle is given by :

`E_k=(\gamma-1)mc^2`

`E_k=(1.032-1)* 1.032 * 10^(-30)* (3* 10^8)^2`

`E_k=2.97* 10^(-15)\ J`

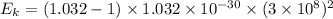
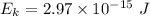
4. The total energy of the particle is :

`E=\gamma mc^2`

`E=1.032* 1.032 * 10^(-30)* (3* 10^8)^2`

`E=9.58* 10^(-14)\ J`

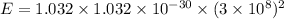
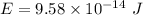
Hence, this is the required solution.