Answer:
Let the dog is walked m miles.
Tonya’s dog-walking service charges a flat rate of $20 per month, plus $3 per mile that each dog is walked.
Equation becomes:
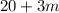
Beth does not charge a monthly fee for her dog-walking service, but she charges $5 per mile that each dog is walked.
Equation becomes:
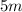
------------------------------------------------------------------------------------------
Part A:
The equation that can be used to find the number of miles m for which Tonya and Beth would charge the same amount.
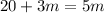
Part B:
Solving for m;
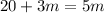
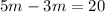
=>
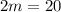
=> m = 10
Hence, both would charge the same amount for 10 miles.