Answer : The final temperature of gas is 266.12 K
Explanation :
According to the Joule-Thomson experiment, it states that when a gas is expanded adiabatically from higher pressure region to lower pressure region, the change in temperature with respect to change in pressure at constant enthalpy is known as Joule-Thomson coefficient.
The formula will be:
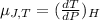
or,
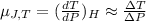
As per question the formula will be:
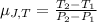 .........(1)
.........(1)
where,
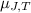 = Joule-Thomson coefficient of the gas =
= Joule-Thomson coefficient of the gas =
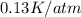
 = initial temperature =
= initial temperature =
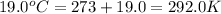
 = final temperature = ?
= final temperature = ?
 = initial pressure = 200.0 atm
= initial pressure = 200.0 atm
 = final pressure = 0.95 atm
= final pressure = 0.95 atm
Now put all the given values in the above equation 1, we get:


Therefore, the final temperature of gas is 266.12 K