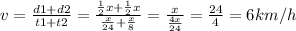Question

½ ofthe distance the horse travelled alonewith velocity 12 km/h And then the poor thing was harnessed into a carriage andtraveled the ½ of the distance withvelocity 4 km/h

What is the average velocitywith which the horse would travel thewhole distance at the same time?

Answer 1

6 km/h

Step-by-step explanation:

For the fist half we must have a distance travelled and the time that this distance was travelled in, we will call this d1, and t1. For the second half we must also have a distance and a time: d2 and t2.

If we say that the total distace was 'x'

`d1=(1)/(2)x`

`d2=(1)/(2)x`

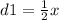
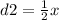
Now, let's find t1 and t2.

for the fist half the horse was traveling at a velocity of 12 km/h, using
`time =(distance)/(velocity)`

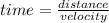
We have for t1:

`t1=((1)/(2)x)/(12km/h) = (x)/(24)`

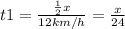
And for t2, since the horse now is traveling at 4 km/h:

`t2=((1)/(2)x)/(4km/h)=(x)/(8)`

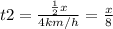
And finally we can find the average velocity, using the formula:

`velocity=(totaldistance)/(totaltime)`

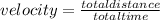
Thus, replacing the values we found:

`v=(d1+d2)/(t1+t2)=((1)/(2)x+(1)/(2)x)/((x)/(24)+(x)/(8)) =(x)/((4x)/(24)) =(24)/(4) =6km/h`