Question

Apply the properties of integer exponents to identify all of the expressions equivalent to 1/8.

A. 2^-3 B. 2^3 C. 1/2^3 D. 2^2 x 2^-5 E. 2^-2 x 2^5

Answer 1

The answers are A , C , D

Explanation:

Lets revise the rule of exponent

*
`a^(n)*a^(m)=a^(m+n)`

*
`(a^(m))/(a^(n))=a^(m-n)`

*
`(1)/(a^(-m))=a^(m)`

*
`((a)/(b))^(-m)=((b)/(b))^(m)`

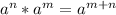
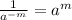
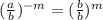
Now lets solve the problem

We need all the expressions equivalent to
`(1)/(8)`

A.

∵
`2^(-3)=(1)/(2^(3))`

∵ 2³ = 8

∴
`2^(-3)=(1)/(8)`

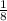
Answer A is equivalent to
`(1)/(8)`

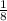
B.

∵ 2³ = 8

Answer B is not equivalent to
`(1)/(8)`

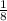
C.

∵ 2³ = 8

∴
`(1)/(2^(3))=(1)/(8)`

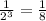
Answer C is equivalent to
`(1)/(8)`

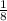
D.

∵
`2^(2)*2^(-5)=2^(2+-5)=2^(-3)`

∵
`2^(-3)=(1)/(2^(3))=(1)/(8)`

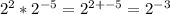
Answer D is equivalent to
`(1)/(8)`

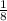
E.

∵
`2^(-2)*2^(5)=2^(-2+5)=2^(3)`

∵ 2³ = 8

Answer E is not equivalent to
`(1)/(8)`

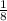
The answers are A , C , D