Question

(a) A photography student borrows RM2 500 to buy a camera. The bank loans this money at a rate of 9 % capitalized monthly. What amount will the student have to reimburse two years later?

Answer 1

The student will have to reimburse 2,991.03 two years later.

Explanation:

This is a compound interest problem:

The compound interest formula is given by:

`A = P(1 + (r)/(n))^(nt)`

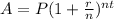
In which A is the amount of money, P is the principal(the initial sum of money), r is the interest rate(as a decimal value), n is the number of times that interest is compounded per unit t and t is the time the money is invested or borrowed for.

In this problem, we have that:

A is the amount the student will have to reimburse two years later.

P is his loan. so
`P = 2,500`

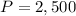
The bank loans this money at a rate of 9 % capitalized monthly. This means that
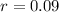`r = 0.09` and
`n = 12`, since the money is compounded monthly, this means, 12 times in a year.

He will have to reimburse two years later, so
`t = 2`

`A = P(1 + (r)/(n))^(nt)`

`A = 2,500(1 + (0.09)/(12))^(24)`

`A = 2,991.03`

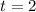
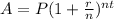
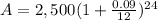
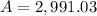
The student will have to reimburse 2,991.03 two years later.