Question

Prove that the product of any two consecutive natural numbers is even.

Answer 1

Answer with Step-by-step explanation:

Let us assume the 2 consecutive natural numbers are 'n' and 'n+1'

Thus the product of the 2 numbers is given by

`Product=n(n+1)\\\\`

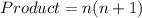
We know that the sum of 'n' consecutive natural numbers starting from 1 is

`S_n=(n(n+1))/(2)\\\\\therefore n(n+1)=2* S_n............(i)`

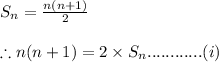
Thus from equation 'i' we can write

`Product=2* S_n`

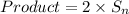
As we know that any number multiplied by 2 is even thus we conclude that the product of 2 consecutive numbers is even.