Answer:
 ≈ -5.1857
≈ -5.1857
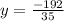 ≈ -5.4857
≈ -5.4857
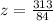 ≈ 3.7262
≈ 3.7262
Explanation:
Rewrite the equation system as:
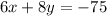
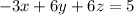
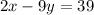
Now, write the system in its augmented matrix form:
![\left[\begin{array}{cccc}6&8&0&-75\\-3&6&6&5\\2&-9&0&39\end{array}\right]](https://img.qammunity.org/2020/formulas/mathematics/college/np6gjvma4n1lzdvjhun22rhkxqngui7jz6.png)
applying row reduction process to its associated augmented matrix:
Swap R1 and R3, and then Swap R1 and R2:
![\left[\begin{array}{cccc}-3&6&6&5\\2&-9&0&39\\6&8&0&-75\end{array}\right]](https://img.qammunity.org/2020/formulas/mathematics/college/ccuwnmhxtwkrewxayif6iir2i7hnl0sqhj.png)
R3+2R1
![\left[\begin{array}{cccc}-3&6&6&5\\2&-9&0&39\\0&20&12&-65\end{array}\right]](https://img.qammunity.org/2020/formulas/mathematics/college/bjqdcb366uho82awj834oo41seeck2y7vt.png)
3R2+2R1
![\left[\begin{array}{cccc}-3&6&6&5\\0&-15&12&127\\0&20&12&-65\end{array}\right]](https://img.qammunity.org/2020/formulas/mathematics/college/e3kvc2qs090x0xikotont5w0fuam17ehqq.png)
15R3+20R2
![\left[\begin{array}{cccc}-3&6&6&5\\0&-15&12&127\\0&0&420&1565\end{array}\right]](https://img.qammunity.org/2020/formulas/mathematics/college/t2hm3wswir78njdrnfezjxjz02n93y03ss.png)
Now we have a simplified system:
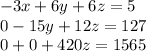

From (3):
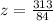 (4)
(4)
Replacing (4) in (2)
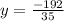 (5)
(5)
Finally replacing (5) and (4) in (1)
