Question

A particle starts from rest at a fixed point 0 and its acceleration is 12t^2-24t+8 m/s^2. Show that the particle is again at rest when it returns to 0 and find its maxi- mum displacement from 0 before it returns. Inte

Answer 1

a) V(2) = 0 The particle is at rest

b) The maximum displacement is 1m

Explanation:

Let's first find velocity and displacement from the given acceleration:

`v(t) = \int\limits {a(t)} \, dt = 4t^3-12t^2+8t`

`x(t) = \int\limits {v(t)} \, dt = t^4-4t^3+4t^2`

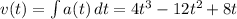
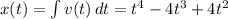
Now we need the instant t1 where x(t1) = 0 and then we evaluate v(t1) to find its velcity and verify if it is 0:

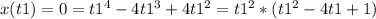`x(t1) = 0 = t1^4-4t1^3+4t1^2 = t1^2 * (t1^2 - 4t1 + 1)` Solving for t, we get:

t1 = 0 and t1 = 2s Now we evaluate v(2):

v(2) = 0m/s It is at rest when it returns to x=0m

Now, for maximum displacement, we need the instant when v(tm) = 0

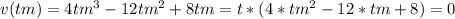`v(tm) = 4tm^3-12tm^2+8tm = t * (4*tm^2 - 12*tm + 8) = 0` Solving for tm:

tm=0; tm = 1 and tm = 2

Since x(0) and x(2) are both 0, we calculate x(1) to find the maximum displacement:

x(1) = 1m