Answer:
Yes. There is 90% confidence the mean will be between ± 1.76% of the sample average.
Explanation:
In this case, where the sample is little and the standard deviation of the population is unknown, the confidence interval (CI) can be expressed as

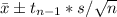
"s" is the standard deviation of the sample and t(n-1) is the critical t-value with (n-1) degrees of freedom. In this case, the degrees of freedom ar (4-1)=3.
The standard deviation is expressed as 1.5% or the mean, that is

Since we have two limits for the mean and a 90% CI, we have 5% of being outside the confidence. Looking up in the table for P(x>t)=0.05 and DF=3, we have
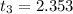
Then the CI is

There is 90% confidence the mean will be between ± 1.76% of the sample average.