Answer:
a) The slope of the tangent line to the graph of the function
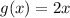 at
at
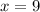 is 2.
is 2.
b) The tangent line is
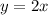 .
.
Explanation:
a) Tho find the slope of the tangent line to the graph of a given function
 at a point
at a point
 we only need to calculate the derivative of
we only need to calculate the derivative of
 at
at
 , i.e.,
, i.e.,
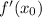 .
.
For the given function
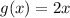 , its derivative is
, its derivative is
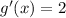 . So, in particular, for
. So, in particular, for
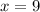 :
:
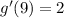 . Thus, the slope of the tangent is 2.
. Thus, the slope of the tangent is 2.
b) The equation of the tangent line of the graph of a function
 at a point
at a point
 is given by the formula
is given by the formula
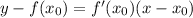 .
.
In this exercise we have the function
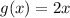 and
and
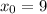 . Then,
. Then,

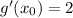 (from the previous answer)
(from the previous answer)
So, the equation of the tangent line is
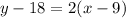
which is equivalent to
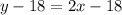
that yields
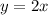 .
.