Answer:
C. 20
Explanation:
The order in which the members are selected is important. The first one selected is the president and the second is the vice president.
So we use the permutations formula to solve this question.
Permutations formula:
The number of possible permutations of x elements from a set of n elements is given by the following formula:
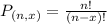
In this problem:
2 people are going to be chosen from a set of 5. So

So the correct answer is:
C. 20