Question

Drag the tiles to the boxes to form correct pairs. Not all tiles will be used. The vertices of polygon ABCD are at A(1, 1), B(2, 3), C(3, 2), and D(2, 1). ABCD is reflected across the x-axis and translated 2 units up to form polygon A′B′C′D′. Match each vertex of polygon A′B′C′D′ to its coordinates. (2, 1) A′ (1, 1) B′ (3, 0) C′ (2, 3) D′ (-3, 4) (2, -1) (-2, 5)

Answer 1

A' (1'1)

B' (2'-1)

C' (3'0)

D' (2'1)

Answer 2

Answer: A'(1,1) , B'(2,-1) , C'(3,0) and D'(2,1)

Explanation:

The transformation rule for reflection across x axis is given by :-

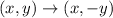`(x,y)\to(x,-y)` (1)

The rule for translation h units above is given by :-

`(x,y)\to(x,y+h)` (2)

Given : The vertices of polygon ABCD are at A(1, 1), B(2, 3), C(3, 2), and D(2, 1). ABCD is reflected across the x-axis and translated 2 units up to form polygon A′B′C′D′.

The coordinates of polygon ABCD after reflection across the x-axis Using (1) :

A(1, 1) → (1,-1)

B(2, 3) → (2,-3)

C(3, 2) → (3,-2)

D(2, 1) → (2,-1)

Now, after translation of 2 units up , the coordinates of polygon A′B′C′D′ using (2 ) will be :

(1,-1) → A'(1,-1+2) = A'(1,1)

(2,-3) → B'(2,-3+2) = B'(2,-1)

(3,-2) → C' (3,-2+2) = C'(3,0)

(2,-1) → D'(2,-1+2) = D'(2,1)

Hence, the answer is A'(1,1) , B'(2,-1) , C'(3,0) and D'(2,1),.