Answer:
Explanation:
We have the equation
 with the initial condition
with the initial condition
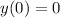 . It is not difficult to notice that this is a linear equation, which has the general expression
. It is not difficult to notice that this is a linear equation, which has the general expression
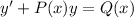 .
.
The solution of this equation is expressed by a general formula:
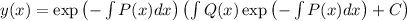 .
.
In the particular case of our equation, we have
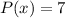
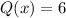 .
.
Then, we must calculate the integrals
 that implies
that implies
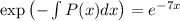 ,
,
and
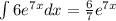
Then,
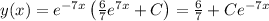 .
.
In order to obtain the value of the constant we substitute the initial condition
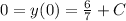 that implies
that implies
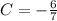
Therefore,
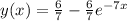 .
.