Question

Solve the equation for all real solutions in simplest form.
z^2 - 12z +9= -3

Answer 1

Solving the equation for all real solutions in simplest form.

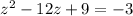`z^2 - 12z +9= -3` we get
`\mathbf{z=10.9\: or\: z=1.1}`

Explanation:

We need to solve the equation for all real solutions in simplest form.

`z^2 - 12z +9= -3`

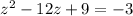
First simplifying the equation:

`z^2 - 12z +9+3= -3+3\\z^2 - 12z +12= 0`

Now, we can solve the equation using quadratic formula:

`z=(-b\pm√(b^2-4ac))/(2a)`

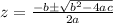
we have a = 1, b=-12 and c=12

Putting values in formula and finding values of x

`z=(-b\pm√(b^2-4ac))/(2a)\\z=(-(-12)\pm√((-12)^2-4(1)(12)))/(2(1))\\z=(12\pm√(144-48))/(2)\\z=(12\pm√(96))/(2)\\z=(12\pm9.8)/(2)\\z=(12+9.8)/(2)\:or\:z=(12-9.8)/(2)\\z=10.9\:or\:z=1.1`

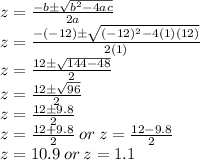
So, we get value of z: z=10.9 or z=1.1

Solving the equation for all real solutions in simplest form.

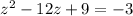`z^2 - 12z +9= -3` we get
`\mathbf{z=10.9\: or\: z=1.1}`