Question

Water is flowing through a 45° reducing pipe bend at a rate of 200 gpm and exits into the atmosphere (P2 = 0 psig). The inlet to the bend is 1 ½ in. inside diameter, and the exit is 1 in. inside diameter. Ignoring losses, calculate the force (magnitude and direction) exerted by the fluid on the bend relative to the direction of the entering stream. Express your answer in units of Newtons. Hint. Use Bernoulli’s equation between the inlet and outlet to determine the upstream pressure P1.

Answer 1

F1=177.88 Newtons

Step-by-step explanation:

Let's start with the Bernoulli's equation:

`P_(1) + (1)/(2)\beta V_(1) ^(2) + \beta gh_(1)  =P_(2) + (1)/(2)\beta V_(2) ^(2) + \beta gh_(2)`

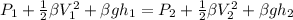
Where:

P is pressure, V is Velocity, g is gravity, h is height and β is density (for water β=1000 kg/m3); at the points 1 and 2 respectively.

From the Bernoulli's equation and assuming that h is constant and P2 is zero (from the data), we have:

`P_(1) + (1)/(2)\beta V_(1) ^(2) = (1)/(2)\beta V_(2) ^(2)`

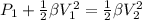
As we know, P1 must be equal to
`(F_(1) )/(A_(1))`, so, replacing P1 in the equation, we have:

`P_(1) = (F_(1))/(A_(1)) = (1)/(2)\beta(V_(2) ^(2) - V_(1) ^(2))`

And

`F_(1) = {A_(1)} ( (1)/(2)\beta(V_(2) ^(2) - V_(1) ^(2)))`

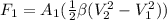
Now, let's find the velocity to replace the values on the expression:

We can express the flow in function of velocity and area as
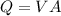`Q = V A`, where Q is flow, V is velocity and A is area. As the same, we can write this:
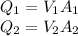`Q_(1) = V_(1) A_(1)\\Q_(2) = V_(2) A_(2)`. In the last two equations, let's clear Velocities.

`V_(1) = (Q_(1))/(A_(1))\\V_(2) = (Q_(2))/(A_(2))`

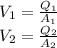
and replacing V1 and V2 on the last equation resulting from Bernoulli's (the one that has the force on it):

`F_(1) = {A_(1)} ( (1)/(2)\beta(((Q_(2))/(A_(2)))^(2) - ((Q_(1))/(A_(1)))^(2)))`

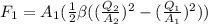
First, we have to consider that from a mass balance, the flow is the same, so Q1=Q2, what changes, is the velocity. Knowing this, let's write the areas, diameters, density and flow on International Units System (S.I.), because the exercise is asking us the answer in Newtons.

`D_(1)=1.5 inches=0.0381 mts\\D_(2)=1 inches=0.0254 mts\\A_(1)=(\pi D_(1)^(2) )/(4)=0.00114mts^(2)\\A_(2)=(\pi D_(2)^(2) )/(4)=0.000507mts^(2)\\\beta=1000 kgs/m^(3)\\Q=200gpm=0.01mts^(3)/seg`

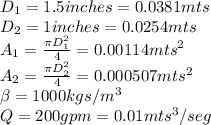
Replacing the respective values in this last expression, we obtain:

F1 = 177.88 N