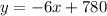Question

The annual yield per lemon tree is fairly constant at 330 pounds per tree when the number of trees per acre is 75 or fewer. For each additional tree over 75​, the annual yield per tree for all trees on the acre decreases by 6 pounds due to overcrowding. Express the yield per​ tree, Y, in​ pounds, as a function of the number of lemon trees per​ acre, x, where x is greater than 75.

Answer 1

We can express the yield per tree as

`y=-6x+780`

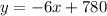
where y: yield per tree and x: number of trees, with x>75.

Explanation:

With this data we have that:

1) The annual yield per lemon tree is 330 pounds per tree when the number of trees per acre is 75

`y(75)=330`

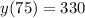
being y the yield per tree.

2) For each additional tree over 75​, the annual yield per tree for all trees on the acre decreases by 6 pounds

`(dy)/(dx)=-6`

being x the number of trees.

If we rearrange and integrate we have

`dy=-6dx\\\\\int dy=-6\int dx\\\\y=-6x+C`

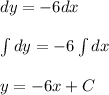
If we use
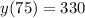`y(75)=330`, we have

`330=-6(75)+C=-450+C\\\\C=330+450=780`

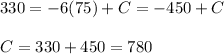
Then we can express the yield per tree as

`y=-6x+780`