Question

You have a mortgage balance of $117,000 that will require you to make 120 more payments of $1,200 , starting next month. Alternatively, you can take out a loan today for $117,000 with an interest rate of 3% APR compounded monthly and pay off the original mortgage. The new loan will require you to make 120 more payments, starting next month. If your investments earn 2.00% APR, compounded monthly, how much will you save in PV terms by taking out the new loan to pay off the original mortgage?

Answer 1

In PV term, we are saving 7,633.33 dollars

Step-by-step explanation:

First, we calculate the PTm of the bank loan:

`PV / (1-(1+r)^(-time) )/(rate) = C\\`

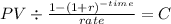
PV $117,000.00

time 120 months

monthly - rate 0.0025 (0.03 annual rate /12 months)

`117000 / (1-(1+0.0025)^(-120) )/(0.0025) = C\\`

C $ 1,129.761

1,200 - 1,129.76 = 70.24

Each month we are saving 70.24 dollars

if this yield 2% we cancalcualte the present value of the savings:

`C * (1-(1+r)^(-time) )/(rate) = PV\\`

C $70

time 120

rate 0.001666667 (0.02/12)

`70.24 * (1-(1+0.0016667)^(-120) )/(0.0016667) = PV\\`

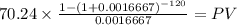
PV $7,633.6663