Answer:
Height is different for both .
The time in air are different for both.
Explanation:
Given that initial velocity is same
Lets take initial velocity = u
One at an angle θ.
other at angle 90° − θ.
Also given that their range are same
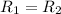
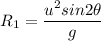
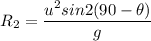
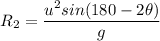
We know that
sin(180° − θ)=sin θ
So
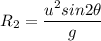
Height in the air
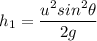
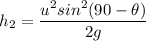
We know that
sin(90° − θ)=cos θ
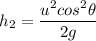
From above we can say that height is different for both .
Time:
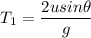
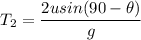
sin(90° − θ)=cos θ
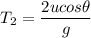
The time in air are different for both.