Question

A wooden crate of mass m = 70 kg slides horizontally off the back of a flatbed truck traveling at 80 km/hr. Determine the kinetic coefficient of friction between the road and the crate if the crate slides (without tumbling) a distance of d = 50 m on the ground before coming to rest. Assume the initial speed of the crate is the same as that of the truck.

Answer 1

0.51

Step-by-step explanation:

m = Mass = 70 kg

g = Acceleration due to gravity = 9.81 m/s²

t = Time taken

u = Initial velocity = 80 km/h = 80/3.6 = 22.22 m/s

v = Final velocity

a = Acceleration

s = Displacement = 50 m

`v^2-u^2=2as\\\Rightarrow a=(v^2-u^2)/(2s)\\\Rightarrow a=(0^2-22.22^2)/(2* 50)\\\Rightarrow a=-4.93\ m/s^2`

`F=ma\\\Rightarrow F=70* 4.93\\\Rightarrow F=345.1`

`F=\mu N\\\Rightarrow 345.1=\mu * 70* 9.81\\\Rightarrow \mu=(345.1)/(70* 9.81)\\\Rightarrow \mu=0.51`

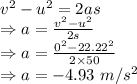
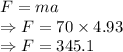
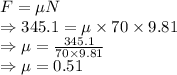
Kinetic coefficient of friction between the road and the crate is 0.51