Answer:
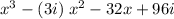 .
.
Explanation:
Notice that the first two factors are in the form
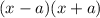 , which is equal to
, which is equal to
 . Start by combining and expanding these two factors:
. Start by combining and expanding these two factors:
Let
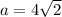 .
.
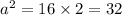 .
.
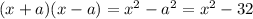 .
.
This expression can now be expressed as
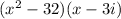 .
.
 stands the unit imaginary number, where
stands the unit imaginary number, where
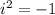 . Unless
. Unless
 is raised to a certain power other than
is raised to a certain power other than
 , it can be treated just like a constant.
, it can be treated just like a constant.
Expand this expression using FOIL:
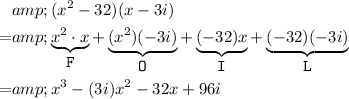 .
.