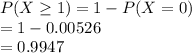Question

Among 13 electrical components exactly 3 are known not to function properly. If 7 components are randomly selected, find the following probabilities: (i) The probability that all selected components function properly. (ii) The probability that exactly 2 are defective. (iii) The probability that at least 1 component is defective.

Answer 1

Explanation:

Given that among 13 electrical components exactly 3 are known not to function properly.

7 components are randomly selected

In this 7 components each component is independent of the other for not to function properly. Also there are two outcomes.

X - no of components not to function properly is Bin with n=7 and

p =
`(3)/(13) =0.2308`

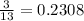
a) The probability that all selected components function properly.

=
`P(x=7) = (1-0.2308)^7\\\\=0.7692^7\\=0.1593`

b) The probability that exactly 2 are defective

= P(x=2) =
`7C2(0.2308)^2(0.7692)^5\\=0.0899`

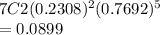
c) The probability that at least 1 component is defective.

=
`P(X\geq 1) =1-P(X=0)\\=1-0.00526\\=0.9947`