Question

In 2002, Tim was twice as old as Sue. In 1997 the sum of their ages was 32. In what year will Sue's age be three-fourths Tim's age​

Answer 1

Year 2030.

Explanation

In 1997, Let Tim's age = X years

In 1997, Let Sue's age = Y years

After 5 years in 2002, Tim's age = (X+ 5) years

After 5 years in 2002, Sue's age = (Y + 5) years

Now, According to question,

X + Y = 32 (sum of their ages) .......(1)

Y = 32 - X

(X + 5) = 2 (Y + 5) .......(2)

Substituting the value of Y in (2)

X + 5 = 2 (32 - X + 5)

X + 5 = 2 (37 - X )

X + 5 = 74 - 2X

3X = 69

X = 69/3 = 23

Now ∵ Y = 32 - X and X = 23

∴ Y = 32 - 23 = 9

So, In 1997, Tim's age = 23 years and Sue's age = 9 years.

Let the year in which Sue's age will be three-fourth times of Tim's age be t.

Sue's age after t years = (9 + t) years.

Tim's age after t years = (23 + t) years

According to question,

`(9 + t) = (3)/(4) *(23 + t)`

`4 (9 + t) = 3 (23 + t)`

`36 + 4t = 69 + 3t`

`4t - 3t = 69 - 36`

`t = 33`

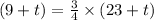
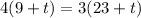
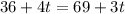
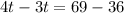
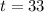
The Year in which Sue's age will be three-fourth times of Tim's age is:

= (1997 + 33) = 2030.