Question

A certain virus infects one in every 200 people. A test used to detect the virus in a person is positive 85% of the time if the person has the virus and 5% of the time if the person does not have the virus. (This 5% result is called a false positive.) a) Find the probability that a person has the virus given that they have tested positive. Round your answer to the nearest tenth of a percent and do not include a percent sign.

Answer 1

Answer: Our required probability is 0.07 i.e. 0.1.

Explanation:

Let A be the event that "the person is infected".

Let B be the event that "the person tests positive".

Probability of person is positive if the person has the virus = 85%

Probability of person is positive if the person does not have the virus = 5%

P(B|A) = 0.85

So, P(A) =
`(1)/(200)=0.005`

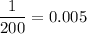
P(B) is given by

`(1)/(200)* 0.85+(199)/(200)* 0.05\\\\=0.00425+0.04975\\\\=0.054`

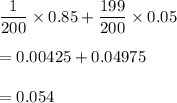
So, We need to find P(A|B) which is given by

`P(A|B)=P(B|A)* (P(A))/(P(B))\\\\P(A|B)=0.85* (0.005)/(0.054)\\\\P(A|B)=0.0787`

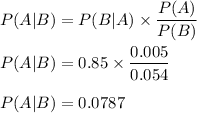
Hence, our required probability is 0.07 i.e. 0.1.