Question

A line has equation y = k, where k is a constant. For which values of k does the line not intersect the circle with equation


`{x}^(2) + 3x + {y}^(2) + 2y - (3)/(4) = 0`
I tried substituting k into y but I don't think that's right because I don't know what to do after that. ​

Answer 1

What you need to do is write the equation of the circle in standard form,

`(x-a)^2+(y-b)^2=r^2`

so that it's easy to identify the two central traits of the circle, its center
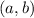`(a,b)` and its radius
`r`.

By completing the square, we have

`x^2+3x+y^2+2y-\frac34=0`

`\left(x^2+3x+\frac94\right)+\left(y^2+2y+1\right)=\frac34+\frac94+1`

`\left(x+\frac32\right)^2+(y+1)^2=4=2^2`

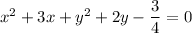
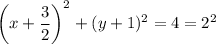
and from this we can tell the circle is centered at
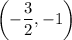`\left(-\frac32,-1\right)` with radius 2.

The line
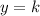`y=k` is horizontal and will intersect the circle whenever
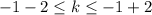`-1-2\le k\le-1+2`, or
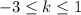`-3\le k\le1`. So the line will not intersect the circle if
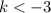`k<-3` or
`k>1`.