Question

In a clinical study, volunteers are tested for a gene that has been found to increase the risk for a disease. The probability that a person carries the gene is 0.1. (a) What is the probability that 4 or more people will have to be tested until 2 of them with the gene are detected? Round your answer to three decimal places (e.g. 0.987). (b) How many people are expected to be tested until 2 of them with the gene are detected? (a) Enter your answer in accordance to the item of the question statement.

Answer 1

probability that 4 or more people P( x≥ 4) = 0.972

expected to be tested = 20

Step-by-step explanation:

we consider here random variable of number is x

and it is tested to detected 2 with gene

so

here x is negative binomial random variable

here probability p = 0.1

and r = 2

then probability mass function of x will be

f(x) =
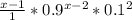`(x-1)/(1) * 0.9^(x-2) * 0.1^2` for x ≥ 2

so now we can calculate as

P( x≥ 4) = 1 - P( x = 3) - P( x = 2)

P( x≥ 4) = 0.972

and

E(x) =
`(2)/(0.1)`

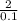
E(x) = 20