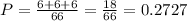Answer:
The probability is 0.2727
Explanation:
There are nCk combinations or ways to take k elements from a group of n elements. So, nCk is calculated as:
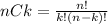
Then, there are 66 ways to select two socks from the 12 that are in the basket. This is calculated as:
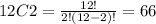
Additionally, if the student match the socks, he have 3 possibilities:
1. He match socks type A
2. He match socks type B
3. He match socks type C
There are 6 ways to match socks type A, 6 ways to match socks type B and 6 ways to match socks type C. This is calculated as:
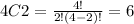
Because the student should select 2 socks type A from the 4 socks type A that are in the basket and it is the same calculation for socks type B and Type C.
Finally, there are 18 possibilities to match the socks, so the probability is calculated as: