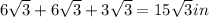Answer:
 in
in
Explanation:
We are given that
Length of one side of quadrilateral=
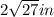
Length of second side of quadrilateral=
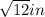
Length of third side of quadrilateral=
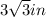
Length of fourth side of quadrilateral=

We have to find the perimeter of the quadrilateral in simplest form.
We know that perimeter of quadrilateral=Sum of length of all sides
Therefore, Perimeter of quadrilateral=
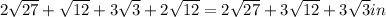
Perimeter of quadrilateral=