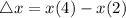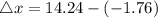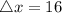Answer and explanation:
Given : The position of an object moving along an x axis is given by
 where x is in meters and t in seconds.
where x is in meters and t in seconds.
To find : The position of the object at the following values of t :
a) At t= 1 s
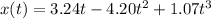
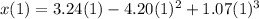

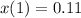
b) At t= 2 s
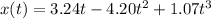
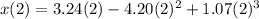

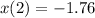
c) At t= 3 s
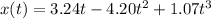
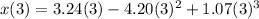

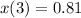
d) At t= 4 s
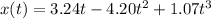
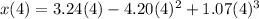
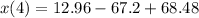
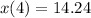
(e) What is the object's displacement between t = 0 and t = 4 s?
At t=0, x(0)=0
At t=4, x(4)=14.24
The displacement is given by,
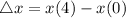
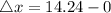
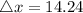
(f) What is its average velocity from t = 2 s to t = 4 s?
At t=2, x(2)=-1.76
At t=4, x(4)=14.24
The average velocity is given by,