Determinați domeniul de definiție al funcției f:D→R, f(x)=(√2-3x)+2

Question

asked Oct 5, 2020 186k views

1 Answer

Wlyles · Answer 1 · 2020-10-11T22:29:36+0000

Answer:

The domain is all real numbers less than or equal to two-third

Explanation:

The question in English is

Determine the domain of the function f :D→R,
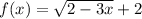
f(x)=√(2-3x)+2

we know that

The radicand of the function must be greater than or equal to zero

so

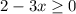
$2-3x \geq 0$

Solve the inequality for x

subtract 2 both sides

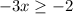
$-3x \geq -2$

Multiply by -1 both sides

$3x \leq 2$

Divide by 3 both sides

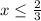
$x \leq (2)/(3)$

The solution is the interval ----> (-∞,2/3]

therefore

The domain is all real numbers less than or equal to two-third