Question

Find the number of seconds in a year, and the fractional error in assuming that 1yr=π×107 sec

Answer 1

There are
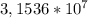`3,1536*10^(7)` seconds in a year.

The fractional error in assuming
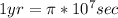`1yr=\pi * 10^(7) sec` is
`3,81*10^(-3)`.

Explanation:

Assuming a year of 365 days (non leap-years), we can found how many seconds are in a year by multiplying seconds in a minute, minutes in an hour, hours in a day and finally days in a year.

`Seconds_(year) = 60(seconds)/(minute) *60(minutes)/(hour)*24(hours)/(day)  *365(days)/(year)=3,1536*10^(7) (seconds)/(year)`

Knowing how many seconds are in a year, we can find the absolute error of the estimation by taking the module of the difference between real value and estimation:

`\varepsilon _(abs) = |real value-estimation|=|3,1536*10^(7) (seconds)/(year)-\pi *10^(7) (seconds)/(year)|=1,2000734641 *10^(5)(seconds)/(year)`

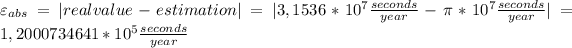
Now that we know the absolute error, we calculate the fractional error by dividing it by the real value:

`\varepsilon _(rel)=(\varepsilon _(abs))/(real value)= (1,2000734641 *10^(5) (seconds)/(year))/(3,1536*10^(7) (seconds)/(year))=3,81 *10^(-3)`

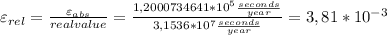
That is a minimal error, close to 0,4% and enough for every day estimations.