Question

Suppose you have 13 tubes of paint. How many distinct color groupings can you make with your paint?

Answer 1

32767 distinct color grouping

Explanation:

Given data:

Total tube is 13

for distinct color, group can be 1,2,3,4,.... 15

For total number of ways we have following relation

`^nC_0 +^nC_1 +^nC_2 + .......^nC_n = 2^n`

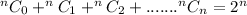
here n = 13 so we have

`^15C_0 +^15C_1 +^15C_2 + .......^15C_(15) = 2^15`

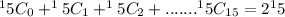
for at least one

`= 2^(15) - 1`

`= 32768 -1`

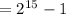
= 32767 distinct color grouping